Technology
Squeezed light from Noisy Labs is quasi-monochromatic continuous-wave laser light at 1064 nm or 1550 nm in a squeezed vacuum state. (Other wavelengths are in preparation). The transverse spatial mode of the beam is close to perfect: TEM00.
In a typical arrangement, the squeezed vacuum state comprises sidebands between 10 kHz and up to more than 30 MHz. The power of the co-propagating monochromatic carrier light can be either 0 or up to a few milliwatts. The guaranteed squeeze factors of up to 10 dB are available.
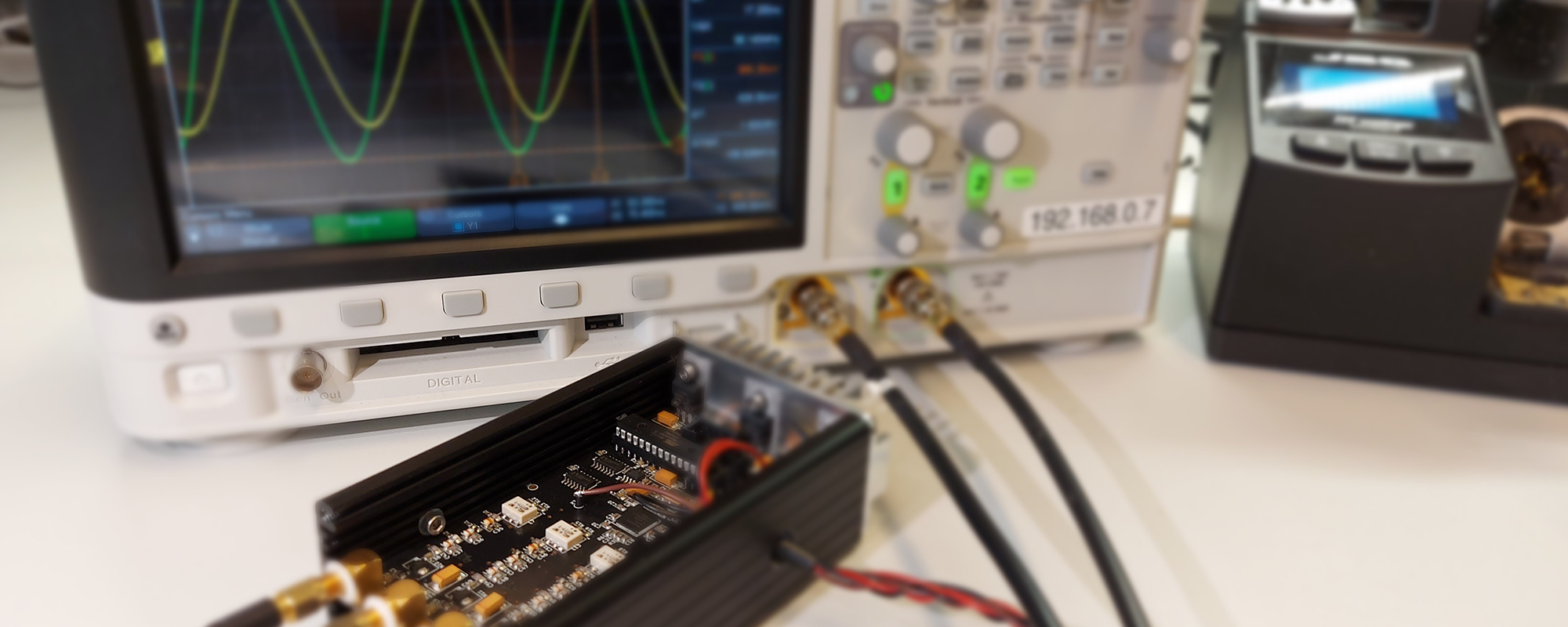
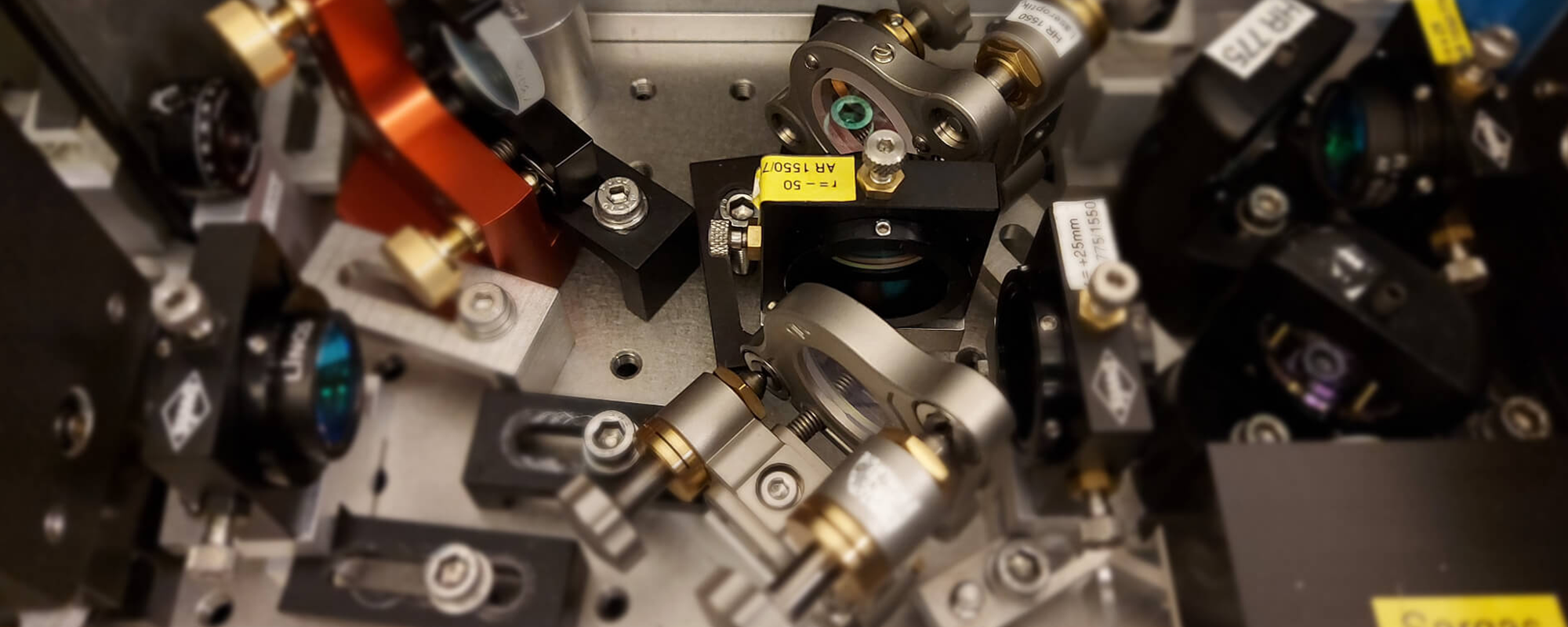
We produce the squeezed light in a non-linear crystal. The crystal is placed in a length-stabilized laser resonator, kept precisely at its optimum temperature just above room temperature and pumped with laser light of half the wavelength (532 nm or 775 nm). Squeezed light is measured with a balanced homodyne detector. Its output voltage is the differential voltage of two photodiodes and is transimpedance amplified.
What is “squeezed light”?
Laser light that is in a squeezed vacuum state is referred to as "squeezed light".
To understand the quantum physics concept itself, you have to imagine a laser beam chopped up into many sections of equal length. These sections are called (longitudinal) "modes". Regardless of the excitation energy, such an ensemble of modes can be in different quantum states. In the best case, a conventional laser beam consists of an ensemble of modes in a coherent state (Glauber state).
Measurements on modes in the coherent state lead to statistically independent photon events. The photon counting statistic follows the Poisson statistic. The result is "photon shot noise", which limits the signal-to-noise ratio of laser measurements.
Measurements on modes in a squeezed vacuum state lead to photon pairs!
In quantum sensing applications, conventional monochromatic laser light carrying a sought-after signal is superimposed with these modes. The result is light with squeezed (reduced) photon shot noise. The signal-to-noise ratio improves. This is exactly what the technological foundation that Noisy Labs builds on achieved between 2010 and 2015 in the GEO600 gravitational wave detector as part of official observation runs. Due to the success at that time, LIGO and Virgo have also been using squeezed light since 2019. Today, all observations of gravitational waves show an improved S/N due to squeezed light.
In sensing, we distinguish between "amplitude-squeezed states" and "phase-squeezed states". The only difference lies in the different phase relationships between the monochromatic carrier light and the squeezed vacuum state. If the signal is an amplitude modulation of the carrier light, the desired phase relation provides amplitude-squeezed states. If the signal is a phase modulation of the carrier light, the desired phase relation provides phase-squeezed states.
The term “squeezed” refers less to the photon statistics than to the quantum uncertainty of the electromagnetic field strength of the light. Light in a squeezed (vacuum) state has an uncertainty of the field strength that is smaller than that of a coherent state for some phases of the wave. To obey Heisenberg's uncertainty relation, a squeezed state must also have phases at which the electric field uncertainty is anti-squeezed, i.e. larger than that of a coherent state [https://en.wikipedia.org/wiki/Squeezed_states_of_light].
In quantum metrology and quantum communication, squeezed vacuum states of light are exploited in a slightly different way. In this case, one is not interested in a signal, but in the decoherence of the squeezed vacuum states due to optical loss. (Only during the measurement are the squeezed vacuum states superimposed with the monochromatic local oscillator beam of the balanced homodyne detector). Decoherence destroys the quantum-correlated photon pairs, which leads to a steep attenuation of the squeezing factor and to a relatively flat attenuation of the anti-squeezing factor.
In metrology, this effect makes it possible to calibrate the quantum efficiency of photosensors.
A "no-go theorem" states that these decoherence effects on the squeezed vacuum states of light cannot be reversed. This fact makes it possible to quantify the amount of eavesdropped light, which is the basis of quantum key distribution and secure communication.
Use Cases for Squeezed Light
There are at least 4 areas of application for squeezed light:
Quantum sensing
Quantum sensing is the most mature application area. GEO600 achieved increased measurement sensitivity with squeezed light during its "Astro-Watch" observations from 2012 to 2015. Since 2019, LIGO and Virgo have been measuring gravitational wave signals with improved S/N due to squeezed light. Squeezing laser light increases your measurement sensitivity when further increasing the light intensity or power is too difficult. 6 dB squeezed light improves the S/N just as if you quadrupled the light power. 10 dB squeezed light is like increasing the light output tenfold. If your biological samples cannot withstand laser light, squeezed laser light may be an option. If you are hesitant to increase light output because of the need for laser safety measures, squeezed light could also be an option.
Quantum metrology
Quantum metrology utilizes the sensitivity of squeezed light to photon losses. With squeezed light, you can absolutely calibrate the quantum efficiency of your photosensors without a source of calibrated spectral radiance.
Quantum key distribution / quantum communication
Quantum key distribution utilizes the sensitivity of squeezed light to photon losses as well as its quantum randomness. Here, the optimal approach is overlapping two squeezed laser beams on a balanced beam splitter. Measurements on the two output beams are private (quantum random) and correlated (entangled).
Optical quantum computing
Continuous-variable optical quantum computing will utilize Gottesman–Kitaev–Preskill (GKP) states to become fault-tolerant, universal and scalable. Squeezed states are the basis on which GKP states can in principle be generated. This is a highly active research topic.